At low pressure the discharge in magnetron sputtering system with the cold cathode is supported basically due to secondary electrons, emitted from the cathode under action of ionic bombardment. Electrons, sputtered from the cathode are accelerated in the field of dark cathode space (DCS) and enter the plasma area with big energies, where they make ionizing collisions with atoms of working gas as long as they loose energy or get to the anode surface.
The estimation by formulas (8.3) shows, that larmor radius of ions rl is more than on two order higher, than larmor radius of electrons, and the magnetic field used in magnetron sputtering systems does not practically influence on the ion trajectory of movement, that is they freely move to the target along trajectories close to rectilinear under action of the electric field. Electrons, moving along complicated trajectories in the field of the magnetic trap, make repeated collisions with atoms of working gas. Hence, it is fair to assume, that electrons play the basic role in formation and maintenance of plasma in magnetron sputtering systems play.
The trajectory of electrons,
emitted with the cathode, is not cycloidal, because of heterogeneity of
electric and magnetic fields. However, for convenience of the analytical
description to a first approximation it is possible to count it close to a
cycloid. As shown in figure 8.1, electron, being
accelerated in the field of dark cathode space in width dK
along a trajectory close to cycloidal, moves away from
the cathode on distancedt and gets in area of
plasma. In the general case dt>dK.
If these values are relative and an electric field is
homogeneous enough in area of DCS,
than dt<<hц,
determined from the formula (8.5), i.e. electron moves
away from the cathode on distance of two larmor radiuses with a speed
.
Ifdt>>dK
electron, receiving energy in DCS,
will move further on larmor circle, and in this case dt
is close to larmor radius, and
(8.7)
where UK — a voltage drop in the field of dark cathode space, V; те – electron mass, kg..
Width of DCS for a case dt=hц=dK is determined by the formula
, (8.8)
where Вk — induction of the magnetic field in the field of dark cathode space, Tl. Width of DCS calculated by the formula (8.8) can be counted maximum possible, because in this case practically all electrons from the cathode move to areas of DCS and do not leave the area of a negative luminescence of plasma where they should effectively ionize the gas. Real width of DCS can be expressed approximately through density of the ionic current by known formula of Child-Lengmur [20, 38, 39]
, (8.9)
where mi – ion mass, kg; ji – density of the ionic current on the cathode, А/m2.
If electron isn’t collide other particle on the way, it comes back to the cathode and can be taken by it. The probability of capture is rather great, because the length of electron free path is greater than length of a cycloid. However, the probability of capture decreases because of wave processes and heterogeneity of magnetic and electric fields, and one consider, that it makes 0.5 [39].
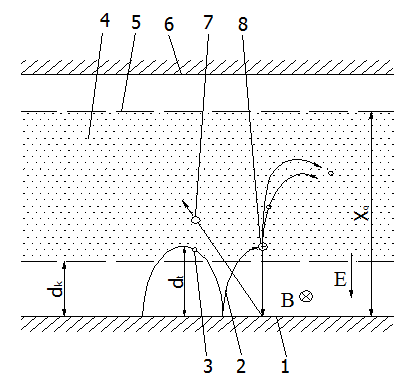
Figure 8.1 The scheme of a discharge gap of magnetron sputtering system: 1 - a cathode - target, 2 - a trajectory of secondary electron, 3 - electron, 4 - plasma, 5 - the conditional anode, 6 - the anode, 7 - the sprayed atom, 8 - an ion.
Entrapped electrons, do not come back to the cathode. They start to make collisions in plasma, as a result of which (and also fluctuations in plasma) they move aside the anode. Having made a little ionizing collisions, electron loses energy and diffuses to the anode. The area within the limits of which electron loses energy, is the area of plasma existence. Border of this area in the discharge of magnetron sputtering systems is the conditional anode.
Уважаемый посетитель!
Чтобы распечатать файл, скачайте его (в формате Word).
Ссылка на скачивание - внизу страницы.