Air to fuel ratio and torque control of spark ignition engines, страница 4

Table 3. Controller parameters.

Initial conditions in integrators , ,.

Simulation results of the system closed by air to fuel and torque controller for different values of external load torques  and reference signals  are presented in figure 1.

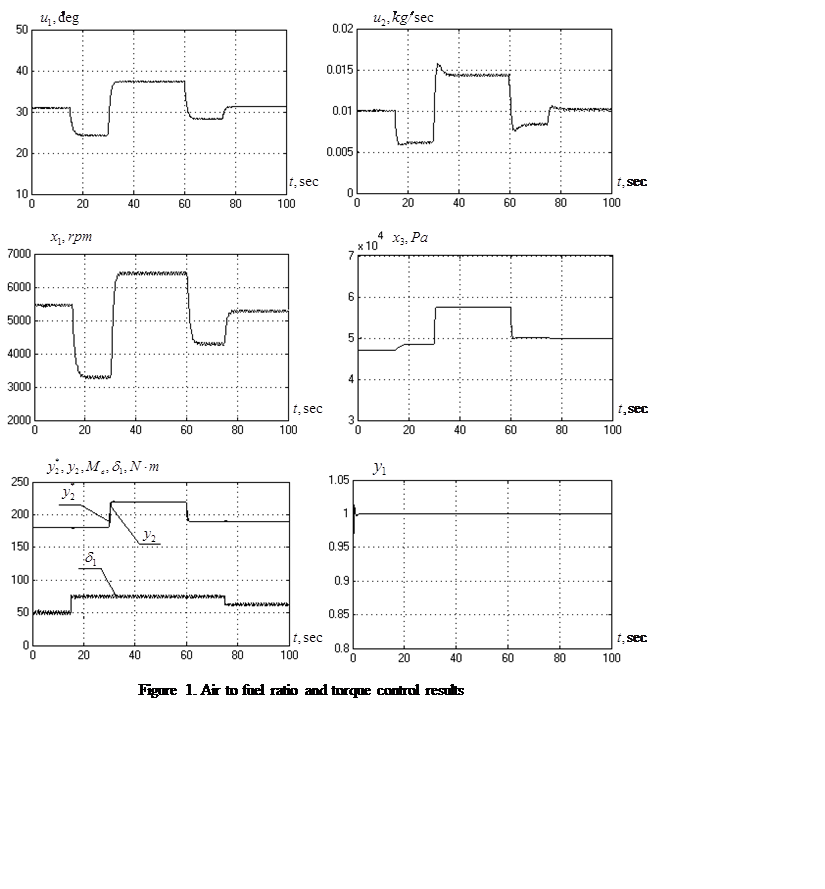Simulation results illustrate perfect work of controller in presence of external load torque disturbance. It necessary to note that output variables do not have an influence on each other, and high operating speed of closed loop system is achieved.

5. Conclusion

Mathematical control oriented model of spark ignition engine is  presented with the purpose of multichannel control synthesis. Algorithm obtained provides stability of closed loop system and allows solving two main problems:

– Adaptive stabilization of air to fuel ratio on the stochiometric value to provide economic consumption of the engine and decrease exhaust emissions.

– Regulation of indicated torque.

The work of the controller is illustrated with the use of simulation. It is shown that the algorithms of coordinated control have perfect quality in absence of noise and external disturbances (besides external load torque).

6. References

[1]. I. Kolmanovsky, M. Druzhinina, J. Sun. Nonlinear torque and air to fuel ratio controller for direct injection stratified charge gasoline engines. Proceedings of AVEC 2000 5^th Int’l symposium on advanced vehicle control. August 22-24, 2000, USA, Ann Arbor, Michigan.

[2]. Christofer H. Onder, Hans Geering. Model-based multivariable speed and air to fuel ratio control of an SI engine. SAE paper 930859.

[3] Ault B.A., Jones V.K., Powell J.D., Franklin G.F. Adaptive air-fuel ration control of a spark ignition engine. SAE paper, No. 940373, 1993.

[4]. Kim Y.W, Rizzoni G and Utkin V. Automotive Engine Diagnosis and Control via Nonlinear Estimation // IEEE Control Systems, October 1998, pp. 84 – 99.

[5]. Dobner D.J. A mathematical engine model for development of dynamic engine control. SAE paper No. 800054, 1980, pp.373–381.

[6]. 8. Simons M.R., Locatelli, Onder C.H., Geering H.P. A Nonlinear Wall-Wetting Model for the Complete Operating Region of a Sequential Fuel Injected SI Engine. SAE Technical Paper 2000-01-1260, 2000.

[7]. Karisson J., Fredriksson J. Cylinder-by-Cylinder Engine Models Vs Mean Value Models for use in Powertrain Control Applications. SAE paper No. 99P-174, 1998.

[8]. Fradkov A.L., Miroshnik I.V., Nikiforov V.O. Nonlinear and adaptive control of complex systems. Dordrecht: Kluwer Academic Publishers, 1999.

[9]. Gerasimov D.N. Adaptive observers with improved parametric convergence. Proceedings of the 10^th International Student Olympiad on Automatic Control (Baltic Olympiad), Russia, Saint-Petersburg, SPbSUITMO pp. 95-100, 2004.

[10].  Bosch.  Automotive book. 5^th Edition. Robert Bosch GmbH, Stuttgart, Federal Republic of Germany , 2000.