3. Dynamic of air pressure in the intake manifold.
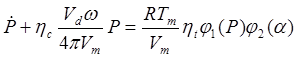 (3)
(3)
where ![]() is the intake manifold volume
is the intake manifold volume ![]() ,
, ![]() is throttle efficiency,
is throttle efficiency,

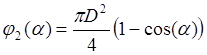
known static
functions, ![]() ,
, ![]() are environment pressure
and temperature,
are environment pressure
and temperature, ![]() is the
specific heat ratio,
is the
specific heat ratio, ![]() is the
throttle angle (
is the
throttle angle (![]() ),
),![]() is the throttle bore
diameter. Equation (3) is derived from Ideal Gas Law equation.
is the throttle bore
diameter. Equation (3) is derived from Ideal Gas Law equation.
4. Fuel to air ratio equation (output)
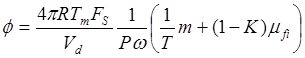 (4)
(4)
where ![]() is the fuel to air ratio.
is the fuel to air ratio.
5. Indicated torque equation [7]
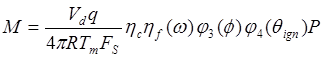 . (5)
. (5)
Thus mathematical model of spark ignition engine can be presented with nonlinear three-order differential equation with two outputs. Let us rewrite it in terms of control-oriented object (plant).
![]() , (6)
, (6)
![]() , (7)
, (7)
![]() , (8)
, (8)
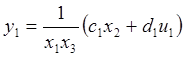 , (9)
, (9)
![]() (10)
(10)
where
control signals: ![]() is the mass flow rate
of fuel injected,
is the mass flow rate
of fuel injected, ![]() is the throttle angle,
is the throttle angle, ![]() is the spark angle (correction signal);
is the spark angle (correction signal);
state variables ![]() is the crank speed,
is the crank speed, ![]() is the mass of fuel film,
is the mass of fuel film, ![]() is the air pressure in the intake
manifold. It is assumed that
is the air pressure in the intake
manifold. It is assumed that ![]() ,
, ![]() are measurable;
are measurable;
regulated variables: ![]() is the normalized fuel
to air ratio in cylinders,
is the normalized fuel
to air ratio in cylinders, ![]() is the indicated
torque.
is the indicated
torque.
![]() is the external load torque (disturbance);
is the external load torque (disturbance);
![]() are corresponding constants which are
calculated with the use of equations (1) –(5).
are corresponding constants which are
calculated with the use of equations (1) –(5).
Problem statement can be formulated with the following expressions:
Air to fuel ratio stabilization
![]() . (11)
. (11)
Indicated torque regulation
![]() (12)
(12)
where ![]() is a desired indicated torque.
is a desired indicated torque.
2. Adaptive air to fuel ratio control
To solve stabilization problem with compensation of uncertain fuel path with the use of adaptive control approach [8, 9].
Thus the model of the air to fuel ratio dynamics is described by
equations (7), (9) where parameters of path dynamic ![]() ,
, ![]() ,
, ![]() are unknown. The posed
control problem will be solved in two steps. First, with the use a special
observer we obtain a suitable parametrization (presentation of regulated
variable in the linear in unknown parameters form) of model (7), (9). Then we
design an adaptive controller via adaptive technique.
are unknown. The posed
control problem will be solved in two steps. First, with the use a special
observer we obtain a suitable parametrization (presentation of regulated
variable in the linear in unknown parameters form) of model (7), (9). Then we
design an adaptive controller via adaptive technique.
To derive a suitable parametrization we use the following filters [5]:
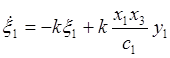 , (13)
, (13)
![]() (14)
(14)
where ![]() is a design parameter. It can be
proved that regulated variable can be presented as [5]
is a design parameter. It can be
proved that regulated variable can be presented as [5]
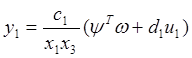 (15)
(15)
where the vector
of unknown model parameters ![]() and vector of
measurable functions
and vector of
measurable functions ![]() are defined as
are defined as
![]() ,
, ![]() . (16)
. (16)
Modifying expressions (15) (16) and introducing control error
![]() , (17)
, (17)
new vectors of controller parameters and measurable functions
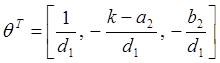 ,
, 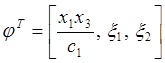 (18)
(18)
we obtain the following parametrized model of the control error
Уважаемый посетитель!
Чтобы распечатать файл, скачайте его (в формате Word).
Ссылка на скачивание - внизу страницы.