


Olga V. Slita
Department of Control Systems and Informatics
Saint-Petersburg State University of Information Technologies, Mechanics and Optics
Kronvercky av., 49, Saint-Petersburg, 197101, RUSSIA
Abstract – conditions of achieving of absolute parametric invariance are formulated. A functional for finding of minimal control ensuring absolute parametric invariance is proposed.
1. INTRODUCTION
In design of control systems desired quality coefficients are given by assigning a spectrum of desired eigenvalues. But when achieving parametric invariance there arises necessity of giving desired geometric properties of designed system by assigning a spectrum of desired eigenvectors. In cases when designer has a opportunity of choosing desired eigenvectors of many possible realizations, preferable choice is the one that is assigned by minimal control costs.
For such a case the given paper proposes a functional that helps evaluate control cost for assigning of desired eigenvectors and find vectors for which parametric invariance is achieved by minimal control.
2. CONDITIONS OF ACHIEVING PARAMETRIC INVARIANCE
Consider the following linear continuous time plant
![]() . (1)
. (1)
where ![]() ,
, ![]() ,
, ![]() are state vector, control and output respectively;
are state vector, control and output respectively; ![]() are nominal component of state matrix, control and
output matrixes:
are nominal component of state matrix, control and
output matrixes: ![]() ;
; ![]() is matrix variation of state matrix,
is matrix variation of state matrix, ![]() ; besides matrix variation is such that pair
; besides matrix variation is such that pair ![]() is controllable.
is controllable.
Let us design control law for plant (1) as
an additive composition of external reference signal ![]() forward control of with matrix
forward control of with matrix ![]() and state
and state ![]() feedback control with matrix
feedback control with matrix ![]() in assumption they are fully measured:
in assumption they are fully measured:
![]() . (2)
. (2)
Plant (1) and control law (2) make a system with the following vector-matrix representation:
![]() ; (3)
; (3)
![]()
![]() . (4)
. (4)
In (3), (4) ![]() is error between reference and output signals and matrixes
is error between reference and output signals and matrixes ![]() and
and ![]() can be represented as
can be represented as
![]() ,
, ![]() , (5)
, (5)
with matrix variation ![]() of state matrix of system satisfying equality
of state matrix of system satisfying equality
![]() , (6)
, (6)
such that ![]() is a Hurwitz matrix.
is a Hurwitz matrix.
Invariance of the system output (and,
hence, the error) regarding uncertainty ![]() can be written in the following form
can be written in the following form
![]() . (7)
. (7)
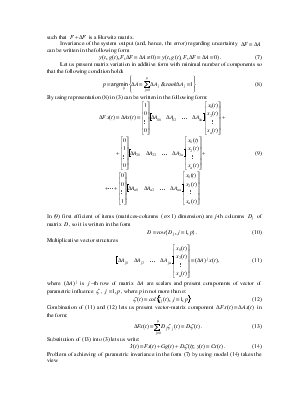
Let us present matrix variation in additive form with minimal number of components so that the following condition holds
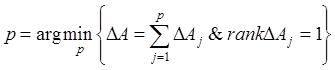 (8)
(8)
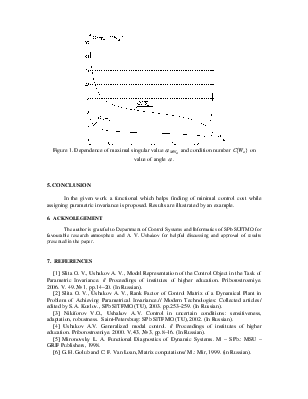
By using representation (8) in (3) can be written in the following form:
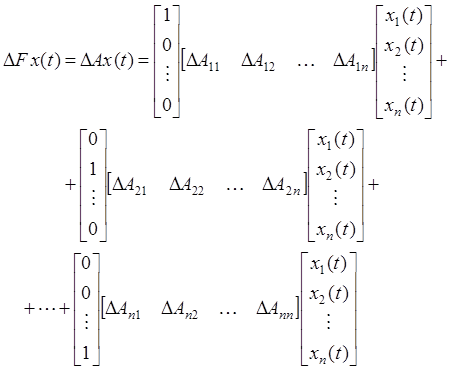 (9)
(9)
In (9) first efficient of items (matrices-columns (![]() ) dimension) are j-th columns
) dimension) are j-th columns ![]() of matrix
of matrix ![]() , so it is written in the form
, so it is written in the form
![]() . (10)
. (10)
Multiplicative vector structures
 , (11)
, (11)
where ![]() is
is ![]() –th row of matrix
–th row of matrix ![]() are scalars and present components of vector of
parametric influence
are scalars and present components of vector of
parametric influence ![]() ,
, ![]() , where p in not more than n:
, where p in not more than n:
![]() (12)
(12)
Combination of (11) and (12) lets us present
vector-matrix component ![]() in the form:
in the form:
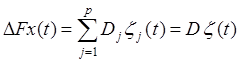 . (13)
. (13)
Substitution of (13) into (3) lets us write:
![]() . (14)
. (14)
Problem of achieving of parametric invariance in the form (7) by using model (14) takes the view
![]() . (15)
. (15)
In terms of Laplace transforms and transfer functions expression (15) takes the form
![]() , (16)
, (16)
where ![]() is Laplace image of reference signal
is Laplace image of reference signal ![]() ,
, ![]() is Laplace image of “parametric” influence
is Laplace image of “parametric” influence
![]() ,
, ![]() is transfer function “reference signal – output of the system”,
is transfer function “reference signal – output of the system”, ![]() is transfer function “parametric influence – output
of the system”.
is transfer function “parametric influence – output
of the system”.
Obviously, this equality holds when
![]() . (17)
. (17)
Let us formulate propositions, containing algebraic conditions of achieving of parametric invariance.
Proposition 1. For system (14) to be parametric invariant in the sense
of condition (16), i.e. for transfer function (matrix) “parametric input ![]() – system output
– system output ![]() ”
”
![]() to be equal to zero, namely the following
expression to hold
to be equal to zero, namely the following
expression to hold
![]() , (18)
, (18)
it is sufficient that
1)
![]() –
– ![]() -th row
of matrix
-th row
of matrix ![]() is eigenvector of the state matrix
is eigenvector of the state matrix ![]() ;
;
2)
![]() belongs to kernel of matrix
belongs to kernel of matrix ![]() so that the following holds
so that the following holds
![]() .
(19)
.
(19)
Proof of proposition 2 can be found in [1].
Proposition 2. For column ![]() of matrix
of matrix ![]() to be eigenvector of matrix
to be eigenvector of matrix ![]() of the system (14) it is sufficient that
vector
of the system (14) it is sufficient that
vector ![]() belongs to image of matrix
belongs to image of matrix ![]() , i.e. the following inclusion holds
, i.e. the following inclusion holds
Уважаемый посетитель!
Чтобы распечатать файл, скачайте его (в формате Word).
Ссылка на скачивание - внизу страницы.