One-Variable Analysis - NORM+UNIF. Analysis Summary

assumption by pressing the alternate mouse button and selecting

Analysis Options.

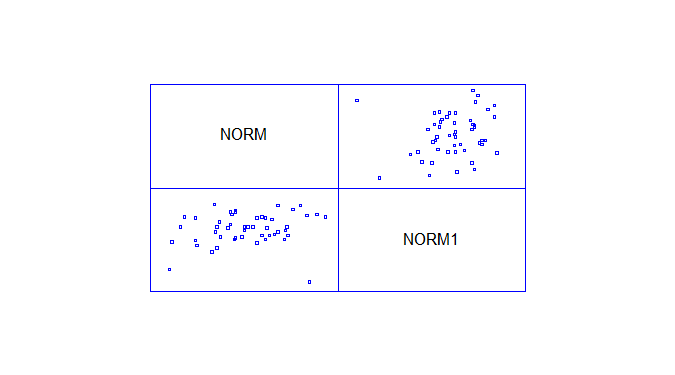
Multiple-Variable Analysis

Analysis Summary

Data variables:

NORM

NORM1

All available data will be used in each calculation.

The StatAdvisor

--------------This procedure is designed to summarize several columns of

quantitative data.  It will calculate various statistics, including

correlations, covariances, and partial correlations.  Also included in

the procedure are a number of multivariate graphs, which give

interesting views into the data.  Use the Tabular Options and

Graphical Options buttons on the analysis toolbar to access these

different procedures.

After this procedure, you may wish to select another procedure to

build a statistical model for your data.  Depending on your goal, one

of several procedures may be appropriate.  Following is a list of

goals with an indication of which procedure would be appropriate:

GOAL: build a model for predicting one variable given values of one of

more other variables.

PROCEDURE: Relate - Multiple regression

GOAL: group rows of data with similar characteristics.

PROCEDURE: Special - Multivariate Methods - Cluster Analysis

GOAL: develop a method for predicting which of several groups new rows

belong to.

PROCEDURE: Special - Multivariate Methods - Discriminant Analysis

GOAL: reduce the number of columns to a small set of meaningful

measures.

PROCEDURE: Special - Multivariate Methods - Factor Analysis

GOAL: determine which combinations of the columns determine most of

the variability in your data.

PROCEDURE: Special - Multivariate Methods - Principal Components

GOAL: find combinations of the columns which are strongly related to

each other.

PROCEDURE: Special - Multivariate Methods - Canonical Correlations

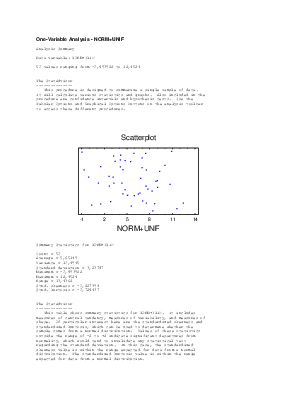
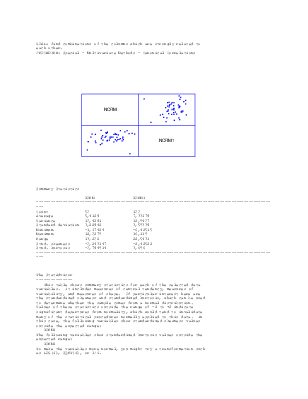
Summary Statistics

NORM                NORM1              

---------------------------------------------------------------------------------------------------Count               50                  100                

Average             5,4129              7,33179            

Variance            10,4291             12,8407            

Standard deviation  3,22942             3,58339            

Minimum             -1,17424            -6,42515            

Maximum             12,0278             16,118             

Range               13,202              22,5431            

Stnd. skewness      -0,243147           -2,42522           

Stnd. kurtosis      -0,794814           3,686              

---------------------------------------------------------------------------------------------------The StatAdvisor

--------------This table shows summary statistics for each of the selected data

variables.  It includes measures of central tendency, measures of

variability, and measures of shape.  Of particular interest here are

the standardized skewness and standardized kurtosis, which can be used

to determine whether the sample comes from a normal distribution.

Values of these statistics outside the range of -2 to +2 indicate

significant departures from normality, which would tend to invalidate

many of the statistical procedures normally applied to this data.  In

this case, the following variables show standardized skewness values

outside the expected range:

NORM1

The following variables show standardized kurtosis values outside the

expected range:

NORM1

To make the variables more normal, you might try a transformation such

as LOG(Y), SQRT(Y), or 1/Y.

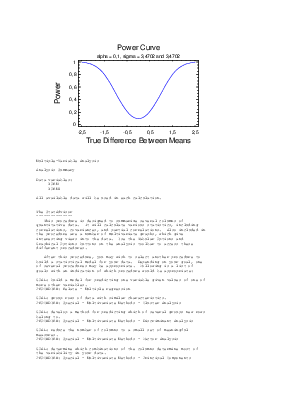
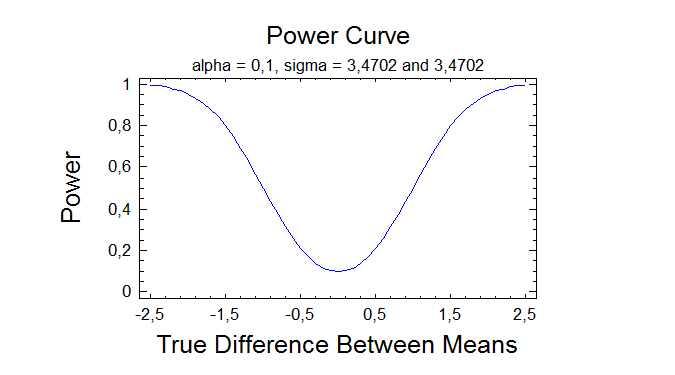
Hypothesis Tests

Hypothesis Tests

---------------Sample standard deviations = 3,22942 and 3,58339

Sample sizes = 50 and 100

95,0% confidence interval for ratio of variances: [0,508453;1,35134]

Null Hypothesis: ratio of variances = 1,0

Alternative: not equal

Computed F statistic = 0,812196

P-Value = 0,423201

Do not reject the null hypothesis for alpha = 0,05.

The StatAdvisor

--------------This analysis shows the results of performing a hypothesis test

concerning the ratio of the standard deviations (sigma1/sigma2) of two

samples from normal distributions.  The two hypotheses to be tested

are:

Null hypothesis:        sigma1/sigma2 = 1,0

Alternative hypothesis: sigma1/sigma2 <> 1,0

Given one sample of 50 observations with a standard deviation of

3,22942 and a second sample of 100 observations with a standard

deviation of 3,58339, the computed F statistic equals 0,812196.  Since

the P-value for the test is greater than or equal to 0,05, the null

hypothesis cannot be rejected at the 95,0% confidence level.  The

confidence interval shows that the values of sigma1/sigma2 supported