Analysis of degeneration of complex dynamic system with human components, страница 3

and such that

,                                                                         (23)

where ,  is vector of fixed factors of frequencies distribution of external multifrequency harmonic influence on -th inputs of system, .          

Matrix equation of Sylvester (22) for a case of external multifrequency harmonic influence is solved for all corner realization  relative to corner realization of matrix  with formation their interval representation.

Interval criterion matrices  that are dispersion matrix  and spectral density matrix  of system’s output for system (8) for a case of external stochastic influence “white noise”  with intensity matrix  can be represented as

,                                                        (24)

,                                                                                                 (25)

where interval matrix  is interval dispersion matrix of state-vector  that satisfies to the matrix algebraic equation of Lyapunov in the form

.                                                                                      (26)

Interval criterion matrices  that are dispersion matrix and spectral density matrix of system’s output for system (8) for a case of external stochastic influence “colored noise”  modeled by output of filter in the following form

; ,                                                                    (27)

stimulated by “white noise”  with intensity matrix , can be determined by (24) and (25), where dispersion matrix  of state vector of system (8) such that

, ,                                                                           (28)

where  is dispersion matrix of compound vector  that satisfies to the matrix algebraic equation of Lyapunov in the form

,                                                                                 (29)

with matrices  and  of compound system, determined in the form

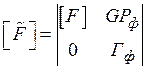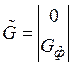, .                                                                                           (30)

Matrix algebraic equation of Lyapunov for a case of external stochastic influence “colored noise” in the form (29) is solved for all corner realization  relative to corner realization of matrix  with formation their interval representation.

V.  CONCLUSION

Analysis of degeneration of complex dynamic system with human components, represented by CDS with interval parameters, can be realized with described technology. If there are human components in a system with corner realization of parameters that is stimulated degeneration of CDS, than such component should be removed out of system and replace to another component with the better parameters.

ACKNOWLEDGMENT

The author would like to thank Prof. A.V. Ushakov for discussions that stimulated her interest in theory of degeneration; his constructive comments that helped improve the paper considerably; useful criticism and advices that have led to the significant improvement of this note.

REFERENCES

[1]  V.O. Nikiforov, A.V. Ushakov. Control in uncertain conditions: sensitiveness, adaptation, robustness. Saint-Petersburg: SPbSU IFMO (TU), 2002 (in Russian).

[2]  E. I. Jury. A literature survey of biocontrol systems. Trans. IEEE (Automatic Control), pp.210-217, July, 1963.

[3]  M.M. Silvestrov, L,M. Koziorov, V.A. Ponomarenko. Automatic control of flying machine with factor of human component. М.: Mashinostroenie, 1986 (in Russian).

[4]  N. A. Dudarenko. Technology for estimation of degeneration of complex dynamic systems systems with frequency separate conditioning values. // Modern technologies: Collection papers / Edited by S.A. Kozlov. SPb: SPbSU IFMO (TU), 2003, pp. 245-252 (in Russian).

[5]  V. V. Voevodin, Yu. A. Smiths, Matrix Calculations. Moscow: Nauka, 1984 (in Russian).

[6]  G. H. Golub and C. F. Van Loan, Matrix Computations, 3th ed. Baltimore, MD: John Hopkins Univ. Press, 1996.

[7]  A.M. Akunova, N.A. Dudarenko, A.V. Ushakov. Problems of degeneration of complex systems: technology of estimation in the case of harmonic and stochastic external influence. // Modern technologies: Collection papers / Edited by S.A. Kozlov. SPb: SPbSU IFMO (TU), 2004 (in Russian).